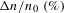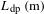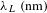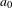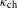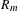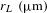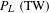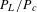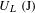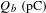Scaling and design of high-energy laser plasma electron acceleration  Download: 846次
Download: 846次
1. Introduction
In this decade, active research has been carried out on the laser plasma acceleration concept[1] in order to achieve high-energy, high-quality electron beams with GeV energy in a cm-scale plasma[2–6], 1%-level energy spread[7], 1-mm-mrad-level transverse emittance[8] and 1-fs-level bunch duration[9], ensuring that the stability of reproduction is as high as that of present high-power ultrashort-pulse lasers[10]. Recently, staged laser plasma acceleration[11, 12] has been successfully demonstrated in conjunction with ionization-induced injection[13–15]. Based on recent results on laser plasma acceleration experiments and large-scale particle-in-cell (PIC) simulations[16–18], design consideration and feasibility studies on applications for high-energy frontier colliders with TeV-range center-of-mass energy have been carried out[19, 20]. In this context, state-of-the-art PW-class lasers allow us to study the feasibility of laser plasma accelerators toward the 10–100-GeV range in a full-scale experiment. For example, a large-scale experiment[21] is proposed to implement the demonstration of 100-GeV electron beam acceleration by means of a laser plasma accelerator driven with a multi-PW laser capable of delivering 3.5-kJ, 500-fs pulses[22]. This capability allows us to explore laser plasma acceleration operated in the entire laser wakefield regime, from the linear regime to the nonlinear bubble regime. Such a large-scale laser plasma accelerator may comprise a gas jet or a short gas cell, which acts as an injector, followed by a long, uniform, low-density plasma or preformed plasma channel (plasma waveguide) that acts as an accelerating medium. In order to implement this project, we need to strongly corroborate design formulas that scale experimental results properly.
We organize the remainder of this paper as follows. Section
2. Overview of laser plasma electron acceleration
Laser-driven plasma-based accelerators have evolved from a groundbreaking concept by Tajima and Dawson[1] into the reality of next-generation particle accelerator technologies. Relativistic electron beams from ultraintense laser plasma interactions can be conceived to be compact particle accelerators, inspiring a wide range of applications of unique particle beam and radiation sources such as THz[23] and betatron x-ray radiation[24]. Furthermore, it is envisaged that laser plasma accelerators will downsize large-scale particle accelerators such as x-ray free-electron lasers[25–28] and high-energy frontier colliders[19, 20] to a realistic extent in both dimensions and costs.
In fact, there has been significant experimental progress in laser wakefield acceleration of electron beams since the incipient experiments on laser wakefield accelerators successfully demonstrated ultrahigh gradient acceleration of the order of 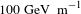 , using chirped pulse amplification lasers with 10-TW class peak power and 1 ps pulse duration[29, 30]. Such experiments are characterized in terms of the self-modulated wakefield regime[31], where the laser power should be higher than the critical power for relativistic self-focusing and the laser pulse duration is longer than the plasma period. In this regime, the laser pulse undergoes temporal intensity modulation and self-guiding through nonlinear interactions with the plasma, so that large-amplitude plasma waves are resonantly excited. Ultimately, wave breakings occur, generating relativistic electrons to be randomly trapped and accelerated by wakefields throughout the acceleration distance. Therefore, electron beams produced from single-stage experiments showed energy spectra with 100% energy spread, as characterized by a Maxwellian distribution with the highest energy tail reaching at most 100 MeV[32]. The energy gain of accelerated electrons should be determined by the acceleration distance, which is restricted due to dephasing of electrons with respect to the correct acceleration phase of the wakefield and due to depletion of the laser pulse energy. For most experiments using a supersonic gas jet, the acceleration distance extends only to a few mm, so that the energy gain is limited to the order of 200 MeV[33].
, using chirped pulse amplification lasers with 10-TW class peak power and 1 ps pulse duration[29, 30]. Such experiments are characterized in terms of the self-modulated wakefield regime[31], where the laser power should be higher than the critical power for relativistic self-focusing and the laser pulse duration is longer than the plasma period. In this regime, the laser pulse undergoes temporal intensity modulation and self-guiding through nonlinear interactions with the plasma, so that large-amplitude plasma waves are resonantly excited. Ultimately, wave breakings occur, generating relativistic electrons to be randomly trapped and accelerated by wakefields throughout the acceleration distance. Therefore, electron beams produced from single-stage experiments showed energy spectra with 100% energy spread, as characterized by a Maxwellian distribution with the highest energy tail reaching at most 100 MeV[32]. The energy gain of accelerated electrons should be determined by the acceleration distance, which is restricted due to dephasing of electrons with respect to the correct acceleration phase of the wakefield and due to depletion of the laser pulse energy. For most experiments using a supersonic gas jet, the acceleration distance extends only to a few mm, so that the energy gain is limited to the order of 200 MeV[33].
For many practical applications of electron beams, quality, stability and controllability of the beam performance such as energy, energy spread, emittance and charge are indispensable in addition to compact and robust features of accelerators. In this context, breakthrough experiments[34–36] have succeeded in producing high-quality electron beams, so-called quasi-monoenergetic beams, with ultrashort pulses, small energy spread and low emittance. Quasi-monoenergetic electron beams have been obtained from the use of ultrashort laser pulses with durations of the order of several tens of femtoseconds and by controlling the plasma density precisely to make the dephasing length long enough to exceed the acceleration distance. Under these conditions, once the plasma electrons expelled by the ponderomotive force (radiation pressure) of the laser pulse form a plasma cavity called a ‘bubble’, then some of them are self-injected into the wakefield by a wave-breaking or restoring force exerted by an ion channel remaining unshielded behind the laser pulse. As a result of beam loading of trapped electrons, the nonlinear wakefield amplitude inside the bubble is reduced below the trapping threshold. Consequently, electrons trapped in the wakefield undergo the processes of acceleration and bunching toward the wave crest to increase their energy and brightness unless the acceleration distance exceeds the dephasing length. This is a scenario of quasi-monoenergetic electron beam acceleration, based on the self-injection mechanism in the bubble regime, which is visually shown by multi-dimensional PIC simulations[37, 38].
Although self-injection is a robust method relying on self-focusing, self-compression of the laser pulse and expansion of the bubble[39] which occur during the propagation of relativistic laser pulses, initially heated (accelerated) electrons with large transverse momentum are injected into nonlinear wakefields that excite betatron oscillation of accelerated electrons due to the strong focusing field. Hence, if the self-injection and the deterioration of beam quality are suppressed, high-quality electron beams can be produced by controlled injection schemes such as colliding optical injection[40, 41], density-transition injection[42] or density down-ramp[43] and ionization-induced injection[13–15] in the quasi-linear regime of wakefields driven by a laser pulse with a moderate intensity. These injection schemes provide us with high-quality electron beam injectors for the front end of multi-stage high-energy accelerators. As a simplest case, two-stage laser plasma acceleration has been successfully demonstrated in combination with ionization-induced injection[11, 12].
For laser plasma acceleration reaching GeV-level energies, it is essential to propagate intense laser pulses over a centimeter-scale distance in underdense plasma. For this purpose, a preformed plasma density channel with a parabolic radial distribution[44] has been developed for guiding a laser beam over many Rayleigh lengths without diffraction which limits the acceleration distance to a few mm in a uniform plasma. Plasma density channels stabilize the propagation of relativistically intense laser pulses under a matched condition, preventing laser plasma nonlinear instabilities, such as filamentation and hosing which often occur in self-guiding[45, 46]. Therefore, the concept of channel-guided laser wakefield accelerators has been a long-standing proposal[47]. Employing a centimeter-scale plasma waveguide, experiments on GeV-level electron acceleration have been carried out with a gas-filled or ablative discharge capillary[2, 7, 8] to demonstrate quasi-monoenergetic electron beams. Experimental progress beyond 1 GeV has been reported in experiments making use of a 100-TW class laser and a centimeter-scale capillary discharge plasma waveguide[4] or a gas cell relying on self-guiding[3]. During the last two decades, a number of laser plasma accelerator experiments have been carried out under various conditions. Comparing these data with theoretical laser wakefield acceleration models, it may be useful to find a correct scaling law capable of predicting energy gain, accelerated electron charge and the required laser and plasma conditions.
3. Energy scaling of laser wakefield acceleration in the relativistic regime
3.1. Propagation of relativistic laser pulses in plasma
The wave equation for the normalized vector potential describing the evolution of a laser pulse with laser wavelength 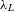 and duration
and duration 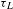 (full width at half maximum, FWHM) in a plasma channel can be written as[48]
(full width at half maximum, FWHM) in a plasma channel can be written as[48]
 (1)
(1) is the vector potential
is the vector potential  of the laser pulse normalized with respect to the electron rest energy
of the laser pulse normalized with respect to the electron rest energy  , satisfying the Coulomb gauge
, satisfying the Coulomb gauge  , and
, and  is the free-space wavenumber along the propagation direction. The (squared) refractive index for linearly polarized electromagnetic waves in the long-pulse limit (
is the free-space wavenumber along the propagation direction. The (squared) refractive index for linearly polarized electromagnetic waves in the long-pulse limit ( ) is given by[49]
) is given by[49] (2)
(2) is the plasma wavenumber evaluated with the unperturbed on-axis density
is the plasma wavenumber evaluated with the unperturbed on-axis density  and the classical electron radius
and the classical electron radius  , and
, and  is the relativistic factor of the laser intensity for linear polarization. In Equation (
is the relativistic factor of the laser intensity for linear polarization. In Equation ( , where
, where  is the laser spot radius and
is the laser spot radius and  is the channel depth. Analysis of the wave equation with the standard paraxial form provides the matched spot radius
is the channel depth. Analysis of the wave equation with the standard paraxial form provides the matched spot radius  under the condition of a beam propagating with a constant spot size
under the condition of a beam propagating with a constant spot size  , i.e.,
, i.e.,  , given by[21]
, given by[21] (3)
(3) is the relativistic factor for a Gaussian laser beam with peak amplitude
is the relativistic factor for a Gaussian laser beam with peak amplitude  and
and  denotes the generalized hypergeometric series of order
denotes the generalized hypergeometric series of order  and class
and class  .
.Under the matched condition that no phase shift of the laser pulse occurs, the group velocity is written as 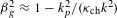 , where a correction factor for the group velocity is defined as[21]
, where a correction factor for the group velocity is defined as[21]
 (4)
(4) .
.3.2. Laser plasma acceleration in the quasi-linear regime
In the linear laser wakefield with the accelerating field 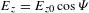 , the equations of the longitudinal motion of an electron with the normalized velocity
, the equations of the longitudinal motion of an electron with the normalized velocity 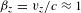 and electron energy
and electron energy 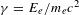 are given by[50]
are given by[50]
 (5)
(5) is the phase of the plasma wave,
is the phase of the plasma wave,  is the non-relativistic wave-breaking field approximately given by
is the non-relativistic wave-breaking field approximately given by 
 ,
,  is the phase velocity
is the phase velocity  of the plasma wave normalized to
of the plasma wave normalized to  , and
, and  is assumed. By integrating Equations (
is assumed. By integrating Equations ( (6)
(6)Setting the initial electron phase 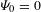 at
at 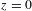 , the maximum energy gain is given by
, the maximum energy gain is given by
 (7)
(7) or
or  . As shown from Equations (
. As shown from Equations ( , the maximum energy gain reaches
, the maximum energy gain reaches  at
at  or
or  . However, electrons undergo both acceleration and focusing only for the phase
. However, electrons undergo both acceleration and focusing only for the phase  . Hence, we define the dephasing length as
. Hence, we define the dephasing length as  . Considering a driving laser pulse of normalized intensity
. Considering a driving laser pulse of normalized intensity  moving in a plasma channel with channel depth
moving in a plasma channel with channel depth  at the group velocity
at the group velocity  with the corresponding relativistic factor
with the corresponding relativistic factor  , where
, where  is the relativistic factor for the group velocity in a uniform plasma and its correction factor
is the relativistic factor for the group velocity in a uniform plasma and its correction factor  is given by Equation (
is given by Equation ( and
and  , respectively. In the limit of
, respectively. In the limit of  ,
,  . In the quasi-linear regime, taking the beam loading effect into account, the maximum accelerating field driven by a Gaussian laser pulse is given by[20]
. In the quasi-linear regime, taking the beam loading effect into account, the maximum accelerating field driven by a Gaussian laser pulse is given by[20] (8)
(8) denotes a factor of accelerating field reduction due to the beam loading effect and
denotes a factor of accelerating field reduction due to the beam loading effect and  is the rms pulse length of the Gaussian temporal profile with the FWHM length
is the rms pulse length of the Gaussian temporal profile with the FWHM length  for
for  .
.3.3. Laser plasma acceleration in the bubble regime
Previous laser plasma acceleration experiments that successfully demonstrated the production of quasi-monoenergetic electron beams with narrow energy spread have been elucidated in terms of self-injection and an acceleration mechanism in the bubble regime[37, 38]. In these experiments, electrons are self-injected into a nonlinear wake, often referred to as a bubble, i.e., a cavity void of plasma electrons consisting of a spherical ion column surrounded by a narrow electron sheath, formed behind the laser pulse instead of a periodic plasma wave in the linear regime. The phenomenological theory of a nonlinear wakefield in the bubble regime describes the accelerating wakefield 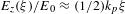 in the bubble frame moving in the plasma with velocity
in the bubble frame moving in the plasma with velocity 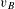 , i.e.,
, i.e., 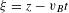 . In the bubble (blowout) regime for
. In the bubble (blowout) regime for 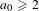 , since the electron-evacuated cavity shape is determined by balancing the Lorentz force of the ion sphere exerted on the electron sheath with the ponderomotive force of the laser pulse, the bubble radius
, since the electron-evacuated cavity shape is determined by balancing the Lorentz force of the ion sphere exerted on the electron sheath with the ponderomotive force of the laser pulse, the bubble radius 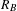 is approximately given as
is approximately given as 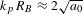 (Ref. [38]). Thus, the maximum accelerating field is obtained as
(Ref. [38]). Thus, the maximum accelerating field is obtained as 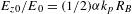 , where
, where  represents a factor that takes into account the difference between the simulation and theoretical estimation, and more significantly the accelerating field reduction due to the beam loading effects.
represents a factor that takes into account the difference between the simulation and theoretical estimation, and more significantly the accelerating field reduction due to the beam loading effects.
In self-guided laser wakefield acceleration, where a driving laser pulse propagates by means of self-channeling, the equations of longitudinal motion of an electron are approximately written as[21]
 (9)
(9) is the longitudinal coordinate of the bubble frame moving at the velocity
is the longitudinal coordinate of the bubble frame moving at the velocity  and taking into account the diffraction at the laser front that etches back at the velocity
and taking into account the diffraction at the laser front that etches back at the velocity  (Ref. [38]). Integrating Equations (
(Ref. [38]). Integrating Equations ( (10)
(10) is the injection energy. Hence, the maximum energy gain is obtained at
is the injection energy. Hence, the maximum energy gain is obtained at  as
as  (11)
(11) for the self-guided bubble regime is given by
for the self-guided bubble regime is given by  (12)
(12) (13)
(13) (14)
(14) is the correction factor of the group velocity for the self-guided pulse in Equation (
is the correction factor of the group velocity for the self-guided pulse in Equation (Table 1. Parameters of experiments on GeV-class laser wakefield acceleration.
|
For a driving laser pulse propagating in a plasma channel, the equations of electron motion are given by setting 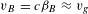 in Equations (
in Equations (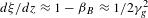 . Hence, the maximum energy gain is
. Hence, the maximum energy gain is
 (15)
(15) is
is  (16)
(16) is given by Equation (
is given by Equation ( (17)
(17) is the rms pulse length.
is the rms pulse length.The matched power 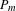 corresponding to the matched spot size
corresponding to the matched spot size 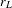 is calculated as
is calculated as
 (18)
(18) is the critical power for the relativistic self-focusing at the plasma density
is the critical power for the relativistic self-focusing at the plasma density  and the required pulse energy is
and the required pulse energy is  .
.3.4. Beam loading effects
In laser wakefield acceleration, an accelerated electron beam induces its own wakefield and cancels the laser-driven wakefield. Assuming the beam loading efficiency 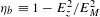 defined by the fraction of the plasma wave energy absorbed by particles of the bunch with the rms radius
defined by the fraction of the plasma wave energy absorbed by particles of the bunch with the rms radius 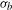 , the beam-loaded field is given by
, the beam-loaded field is given by 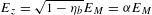 , where
, where 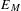 is the accelerating field without beam loading, given by
is the accelerating field without beam loading, given by 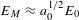 for the bubble regime
for the bubble regime 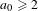 . Thus, the loaded charge is calculated as[20]
. Thus, the loaded charge is calculated as[20]
 (19)
(19) for accelerating charge
for accelerating charge  in the operating plasma density
in the operating plasma density  is obtained by solving the equation
is obtained by solving the equation  (20)
(20) is defined as
is defined as  (21)
(21) (22)
(22) , the field reduction factor becomes
, the field reduction factor becomes  and contrarily, for
and contrarily, for  ,
,  .
.3.5. Comparison with experimental results on GeV-class electron beams
Table  (Refs. [3, 5, 6, 11, 12, 51, 52]) and a channel-guided laser pulse with
(Refs. [3, 5, 6, 11, 12, 51, 52]) and a channel-guided laser pulse with 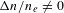 (Refs. [2, 4, 7, 8, 53]). Since the maximum energy gain scales as
(Refs. [2, 4, 7, 8, 53]). Since the maximum energy gain scales as 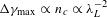 for a given
for a given 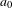 , most previous experiments have employed chirped pulse amplification lasers with wavelength
, most previous experiments have employed chirped pulse amplification lasers with wavelength  and pulse duration
and pulse duration 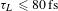 , except for the case of Ref. [5], where a PW-class laser with wavelength
, except for the case of Ref. [5], where a PW-class laser with wavelength 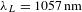 and
and 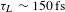 was employed. The validity of the energy scaling formulas (Equations (
was employed. The validity of the energy scaling formulas (Equations (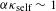 or
or 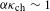 .
.
For self-guided laser wakefield acceleration, the multi-GeV acceleration results reported in Refs. [5, 6] provide us with informative examples for testing the energy scaling formula (Equation ( is focused onto a
is focused onto a 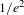 spot radius of
spot radius of 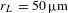 , producing
, producing 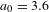 at the entrance of a 7-cm long gas cell with
at the entrance of a 7-cm long gas cell with 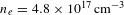 . The accelerated electron beam has a quasi-monoenergetic peak at 2.0 GeV with a relative energy spread of 10% (FWHM), containing a total charge of 540 pC in a bunch. In this case, from Equations (
. The accelerated electron beam has a quasi-monoenergetic peak at 2.0 GeV with a relative energy spread of 10% (FWHM), containing a total charge of 540 pC in a bunch. In this case, from Equations (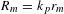 and the correction factor of the group velocity
and the correction factor of the group velocity 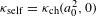 are
are 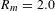 and
and  , respectively, at
, respectively, at 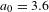 . Thus, since the matched spot radius is
. Thus, since the matched spot radius is 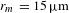 , the laser pulse undergoes self-focusing after propagating through the gas cell. Using Equations (
, the laser pulse undergoes self-focusing after propagating through the gas cell. Using Equations ( , the field reduction factor
, the field reduction factor  is calculated as
is calculated as 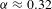 (
(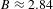 ). From Equation (
). From Equation (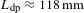 , while from Equation (
, while from Equation (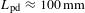 . From Equation (
. From Equation (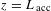 is estimated as
is estimated as
 (23)
(23) is the injection beam energy. For
is the injection beam energy. For  , the beam energy is evaluated to be
, the beam energy is evaluated to be  . This estimate is in good agreement with the measured beam energy of
. This estimate is in good agreement with the measured beam energy of  [5], taking into account the field reduction factor
[5], taking into account the field reduction factor  due to beam loading.
due to beam loading.
Fig. 1. A comparison of measured electron beam energies in laser wakefield acceleration with the energy scaling as a function of the operating plasma density for (a) the self-guided case in the bubble regime at laser wavelengths of 800 nm (solid line) and 1057 nm (dashed line) and (b) the channel-guided case in both the quasi-linear regime (dashed line) and the bubble regime (solid line). The experimental data are plotted with filled squares for  and the open square for
and the open square for 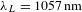 in (a), and with filled circles for
in (a), and with filled circles for 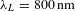 in (b).
in (b).
![Electron beam energy spectra obtained from the experiment[6], where a 212-TW, 60-fs laser pulse is focused on a spot radius of producing at the entrance of a gas jet for three cases consisting of (a) a 4-mm long single stage with , (b) a 10-mm long single stage with and (c) two stages comprising a 4-mm long injector with and a 10-mm long accelerator with .](/richHtml/hpl/2015/3/1/01000e10/img_2.gif)
Fig. 2. Electron beam energy spectra obtained from the experiment[6], where a 212-TW, 60-fs laser pulse is focused on a  spot radius of
spot radius of 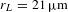 producing
producing 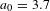 at the entrance of a gas jet for three cases consisting of (a) a 4-mm long single stage with
at the entrance of a gas jet for three cases consisting of (a) a 4-mm long single stage with 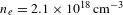 , (b) a 10-mm long single stage with
, (b) a 10-mm long single stage with 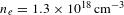 and (c) two stages comprising a 4-mm long injector with
and (c) two stages comprising a 4-mm long injector with  and a 10-mm long accelerator with
and a 10-mm long accelerator with 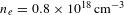 .
.
In Ref. [6], a 212-TW, 60-fs laser pulse is focused on a  spot radius of
spot radius of 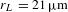 , producing
, producing  at the entrance of a gas jet for three cases consisting of a 4-mm long single stage with
at the entrance of a gas jet for three cases consisting of a 4-mm long single stage with  , a 10-mm long single stage with
, a 10-mm long single stage with  , and two stages comprising a 4-mm long injector with
, and two stages comprising a 4-mm long injector with 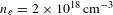 and a 10-mm long accelerator with
and a 10-mm long accelerator with 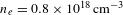 . As shown in Figure
. As shown in Figure 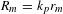 and the correction factor of the group velocity
and the correction factor of the group velocity 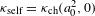 are
are 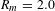 and
and 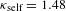 , respectively, at
, respectively, at 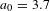 . Since the matched spot radius is
. Since the matched spot radius is 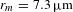 for the 4-mm single-stage case,
for the 4-mm single-stage case, 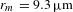 for the 10-mm single-stage case and
for the 10-mm single-stage case and  in the injector jet and
in the injector jet and 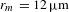 in the accelerator jet for the two-stage case, respectively, it is inferred that a laser pulse with a focused spot radius of
in the accelerator jet for the two-stage case, respectively, it is inferred that a laser pulse with a focused spot radius of 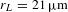 is initially self-focused down to the matched spot radius. Using Equations (
is initially self-focused down to the matched spot radius. Using Equations (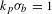 , the field reduction factor
, the field reduction factor  is calculated as
is calculated as 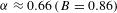 for the 4-mm single-stage case,
for the 4-mm single-stage case, 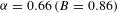 for the 10-mm single-stage case and
for the 10-mm single-stage case and 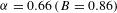 in the injector/
in the injector/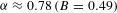 in the accelerator for the two-stage case. From Equation (
in the accelerator for the two-stage case. From Equation (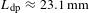 for the 4-mm single-stage case,
for the 4-mm single-stage case, 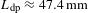 for the 10-mm single-stage case and
for the 10-mm single-stage case and 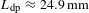 in the injector/
in the injector/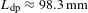 in the accelerator for the two-stage case. The pump depletion length due to pulse-front erosion is
in the accelerator for the two-stage case. The pump depletion length due to pulse-front erosion is 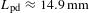 for the 4-mm single-stage case,
for the 4-mm single-stage case, 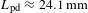 for the 10-mm single-stage case and
for the 10-mm single-stage case and  in the injector/
in the injector/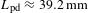 in the accelerator for the two-stage case. From Equation (
in the accelerator for the two-stage case. From Equation (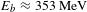 with an effective acceleration length of
with an effective acceleration length of 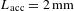 for the 4-mm single-stage case and
for the 4-mm single-stage case and 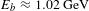 with
with 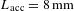 for the 10-mm single-stage case. For the two-stage case, the output energy of the injector is
for the 10-mm single-stage case. For the two-stage case, the output energy of the injector is  with
with 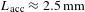 and the output energy of the accelerator stage reaches
and the output energy of the accelerator stage reaches 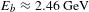 with
with 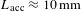 , assuming that the injection energy is
, assuming that the injection energy is 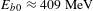 and the focused spot size at the entrance of the accelerator stage is decreased to
and the focused spot size at the entrance of the accelerator stage is decreased to 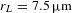 due to self-focusing in the injector stage, increasing the normalized vector potential up to
due to self-focusing in the injector stage, increasing the normalized vector potential up to 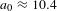 . In this experiment, most of the charge produced in the injector is injected into the accelerator stage, while the large energy spread is attributed to the fact that the accelerator length is shorter than the dephasing length at which the energy compression takes place in the phase space as well as the maximum energy.
. In this experiment, most of the charge produced in the injector is injected into the accelerator stage, while the large energy spread is attributed to the fact that the accelerator length is shorter than the dephasing length at which the energy compression takes place in the phase space as well as the maximum energy.
4. Design of 10-GeV-level laser plasma accelerators
At present, the most near-term prospects for 10-GeV-level laser plasma acceleration are confidently given by the scaling and methods described in the previous section. Here, we consider design examples of laser plasma accelerators capable of delivering 10-GeV electron beams with bunch charges of 160 pC (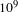 electrons per bunch) for three cases: a self-guided laser plasma accelerator in the bubble regime with
electrons per bunch) for three cases: a self-guided laser plasma accelerator in the bubble regime with 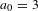 , a channel-guided laser plasma accelerator in the bubble regime with
, a channel-guided laser plasma accelerator in the bubble regime with 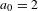 and a channel-guided laser plasma accelerator in the quasi-linear regime with
and a channel-guided laser plasma accelerator in the quasi-linear regime with 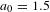 . For all three cases, we present design parameters for the laser and plasma for a driving laser wavelength of 800 nm. Table
. For all three cases, we present design parameters for the laser and plasma for a driving laser wavelength of 800 nm. Table 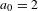 and a beam loading charge of 300 pC by comparison with the results of 3D PIC simulation from the Lorentz-boosted frame OSIRIS code[16]. The design parameters for the 40-GeV laser plasma accelerator are in good agreement with the PIC simulation results in terms of the operating plasma density, the accelerator length, i.e., the dephasing length, the matched spot radius and the matched power. The design formulas we used to evaluate these parameters are described in the following.
and a beam loading charge of 300 pC by comparison with the results of 3D PIC simulation from the Lorentz-boosted frame OSIRIS code[16]. The design parameters for the 40-GeV laser plasma accelerator are in good agreement with the PIC simulation results in terms of the operating plasma density, the accelerator length, i.e., the dephasing length, the matched spot radius and the matched power. The design formulas we used to evaluate these parameters are described in the following.
Table 2. Design parameters for 10-GeV-level laser plasma accelerators in comparison with the results of the 3D PIC simulation[16]. Case A stands for the self-guided case in the bubble regime, designed by the formulas given in Section 4.1 , case B for the self-guided case in the bubble regime, designed by the formulas given in Section 4.2 , case C for the channel-guided case in the quasi-linear regime, designed by the formulas given in Section 4.3 , and case D for the self-guided case in the bubble regime at 40 GeV, designed by the formulas given in Section 4.1 .
|
4.1. Self-guided laser plasma accelerator in the bubble regime
For a given energy gain 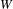 , the operating plasma density is determined from Equation (
, the operating plasma density is determined from Equation (
 (24)
(24) (25)
(25) . The dephasing length should be less than the pump depletion length, i.e.,
. The dephasing length should be less than the pump depletion length, i.e.,  . Therefore, the pulse length is set to be
. Therefore, the pulse length is set to be  .
.  (26)
(26) (27)
(27) is the dimensionless matched spot radius given by Equation (
is the dimensionless matched spot radius given by Equation ( . The matched power is calculated as
. The matched power is calculated as  (28)
(28) (29)
(29) for loading charge
for loading charge  up to a given energy
up to a given energy  is obtained by solving the equation
is obtained by solving the equation  (30)
(30) is given as
is given as  (31)
(31)4.2. Channel-guided laser plasma accelerator in the bubble regime
The operating plasma density is determined by
 (32)
(32) (33)
(33) (34)
(34) is the rms pulse length. The requirement for the accelerator length
is the rms pulse length. The requirement for the accelerator length  bounds the minimum pulse duration as
bounds the minimum pulse duration as  (35)
(35)The matched spot radius becomes
 (36)
(36) is the dimensionless matched spot radius given by Equation (
is the dimensionless matched spot radius given by Equation ( (37)
(37) (38)
(38) is determined from the coefficient
is determined from the coefficient  , given by
, given by  (39)
(39)4.3. Channel-guided laser plasma accelerator in the quasi-linear regime
For a given 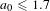 , the pulse duration is given by
, the pulse duration is given by
 (40)
(40) (41)
(41) can be set to be
can be set to be  (42)
(42) is given by Equation (
is given by Equation ( (43)
(43) is the dimensionless matched spot radius for a given
is the dimensionless matched spot radius for a given  and
and  , given by Equation (
, given by Equation ( (44)
(44) (45)
(45) is determined from the coefficient
is determined from the coefficient  , given by
, given by  (46)
(46)5. Conclusion
We have provided an overview of recent progress in laser plasma accelerators from the perspective of experiments on the production of GeV-level electron beams, and scaling formulas to describe energy gain for a self-guided laser plasma accelerator in the bubble regime (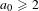 ), a channel-guided laser plasma accelerator in the bubble regime (
), a channel-guided laser plasma accelerator in the bubble regime (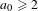 ) and a channel-guided laser plasma accelerator in the quasi-linear regime (
) and a channel-guided laser plasma accelerator in the quasi-linear regime ( ). Although most previous experiments have been focused on electron injection into the plasma bubble and the production of high-quality electron beams with small energy spread and emittance, employing a millimeter-scale gas jet, recent experimental results beyond 1-GeV acceleration allow us to test the scaling formulas in depth, which are necessary for the design of the operating parameters of laser plasma accelerators to satisfy requirements such as energy gain and beam charge. Taking account of the group velocity correction factor
). Although most previous experiments have been focused on electron injection into the plasma bubble and the production of high-quality electron beams with small energy spread and emittance, employing a millimeter-scale gas jet, recent experimental results beyond 1-GeV acceleration allow us to test the scaling formulas in depth, which are necessary for the design of the operating parameters of laser plasma accelerators to satisfy requirements such as energy gain and beam charge. Taking account of the group velocity correction factor 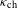 in the propagation of laser pulses with relativistic intensity, characterized by
in the propagation of laser pulses with relativistic intensity, characterized by 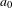 , through plasma channels, including initially uniform plasma with
, through plasma channels, including initially uniform plasma with 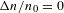 and a preformed plasma channel with
and a preformed plasma channel with 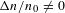 , provides the correct accelerator length equal to the dephasing length as well as the proper operating plasma density. Meanwhile, we found that the accelerating field reduction factor
, provides the correct accelerator length equal to the dephasing length as well as the proper operating plasma density. Meanwhile, we found that the accelerating field reduction factor  due to beam loading can be properly evaluated by applying the resultant scaling formulas to recent experimental results for multi-GeV laser plasma accelerators[5, 6], leading to self-consistent design parameters for the driving laser pulse achieving the requirement of beam energy and charge. Independently, we confirmed that the accelerating field reduction factor
due to beam loading can be properly evaluated by applying the resultant scaling formulas to recent experimental results for multi-GeV laser plasma accelerators[5, 6], leading to self-consistent design parameters for the driving laser pulse achieving the requirement of beam energy and charge. Independently, we confirmed that the accelerating field reduction factor  can be obtained from the simple analytic formulas Equations (
can be obtained from the simple analytic formulas Equations (
[1]
[2]
[3]
[7]
[8]
[9]
[10]
[12]
[13]
[14]
[16]
[18]
[19]
[20]
[21]
[22]
[23]
[24]
[25]
[26]
[27]
[29]
[30]
[32]
[33]
[34]
[35]
[36]
[37]
[38]
[39]
[40]
[41]
[42]
[43]
[44]
[45]
[47]
[48]
[49]
[50]
[53]
Article Outline
Kazuhisa Nakajima, Hyung Taek Kim, Tae Moon Jeong, Chang Hee Nam. Scaling and design of high-energy laser plasma electron acceleration[J]. High Power Laser Science and Engineering, 2015, 3(1): 01000e10.
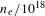

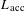
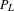
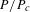
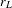
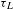
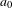
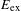
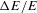
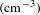
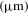
 100
100 2.5
2.5 3
3 5
5 25
25 30
30 0.8
0.8 10
10 30
30 5
5 5
5 5
5 5
5 5
5 5
5 50
50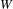 (GeV)
(GeV)