1 Introduction

Phase measurement is very important in optics shape measurement. For its non-contact operation, high precision, high speed and other measurement advantages, it is widely used in production, national defense, scientific research and other fields^[1-6]. Numerous scholars have studied phase measurement and obtained good results^[1-3]. For example, in 2020, to better measure isolated targets with complex surfaces, Cai et al. improved the measurement method of the absolute phase by using half-cycle correction and proposed a new gray coding method^[1]. In 2021, in order to optimize phase measurement, Peng et al. proposed a sine fringe generation technique for three-dimensional shape measurement^[2]. In 2022, to improve the reliability of tri-frequency time phase unwrapping, Hou et al. proposed a method using spatiotemporal tri-frequency time phase unwrapping^[3].

Due to the nonlinear effect of the measurement system, phase measurement will be adversely affected. To reduce or even eliminate the nonlinear effect influence and improve measurement accuracy, some scholars have studied it and made some good achievements^[7-11]. In 2013, to correct the system’s gamma nonlinearity, Xiao et al. presented a single orthogonal sinusoidal grating used in phase measurement, where the fringe gained by projecting the grating has good sinusoidal properties and can decrease the phase sinusoidal error^[7]. In 2015, to decrease the phase measurement error induced by the system’s gamma nonlinearity, Xu et al. corrected the fringe using the system response function^[8]. In 2021, for the three-step phase-shifting profilometry based nonlinear effect, Yang et al. proposed a method to reduce phase error with a three-to-three deep learning framework^[9].

In this paper, the causes of the nonlinear effect influencing fringe intensity in the measurement system and its influence are analyzed in detail. After the nonlinear effect is reduced, the advantages of the dual-frequency grating method are used to gain better phase information of the measured object fringe image, and analyze its principle. To verify the principle analysis, a computer simulation and a practical experiment are executed, whose results show that the principle is correct.

2 Principle analysis

2.1 Nonlinear effect of system in phase measurement

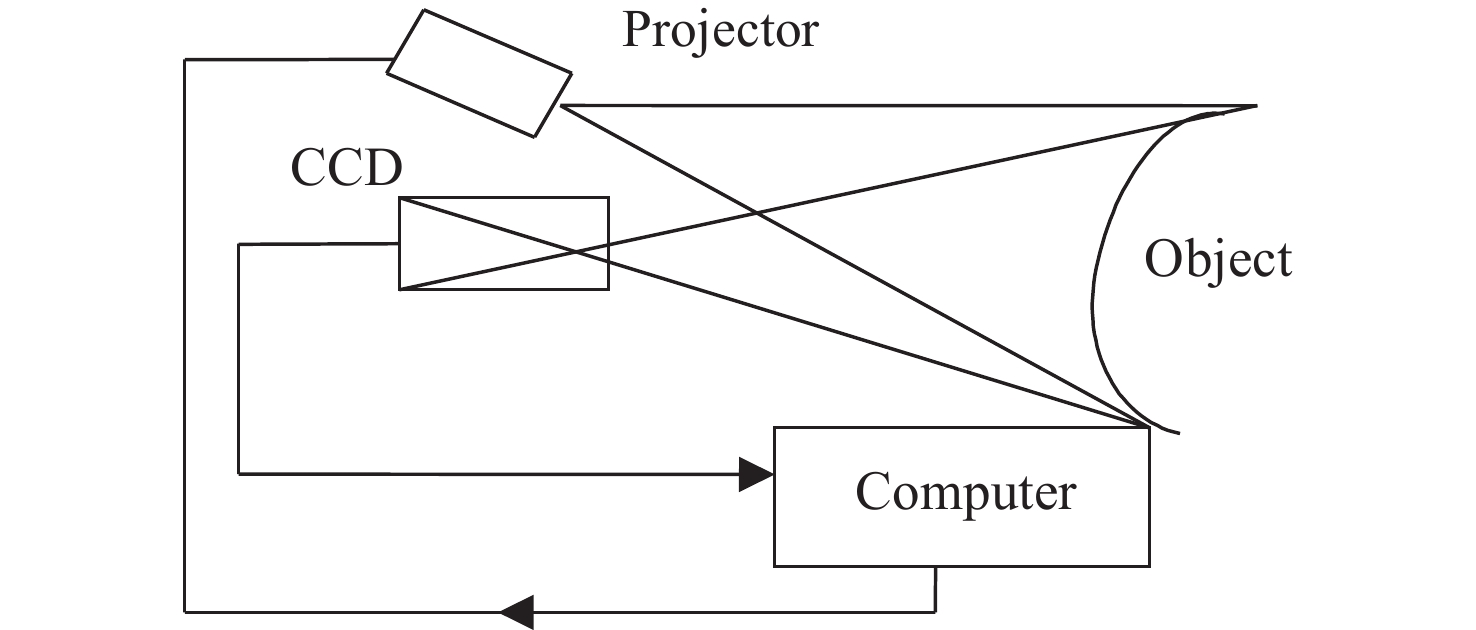
Phase measurement is very common in optics shape measurement. A diagram of the system’s structure and its corresponding parameters are described in Fig. 1 of the literature [12].

图 1.

Fig. 1. Simulated phase

下载图片 查看所有图片

In ideal conditions, the light intensity of deformed fringe outputed from the projector system and that inputed to CCD^[13] is linear. The light intensity image of the sinusoidal fringe from the projector system output is gained by CCD, as shown in the following expression:

$ g(x,y) = a(x,y) + b(x,y)\cos [2{\text{π}} {f_0}x + \phi (x,y)]\quad, $ (1)

where $ a(x,y) $ indicates the background light field of the stripe, $ b(x,y) $ indicates stripes contrast, $ {f_0} $ indicates grating fundamental frequency, and $ \phi (x,y) $ indicates phase information.

Fourier transform is executed to the light intensity of the fringe along the $ x $ axis. In the frequency domain, the zero-order spectrum components are eliminated by using the $ {\text{π}} $ phase-shift technology^[14]. The spectra expression containing the height information $ h(x,y) $ of the object can be gained as follow:

$ G({f_x},{f_y}) = Q({f_x} - {f_0},{f_y}) + {Q^*}({f_x} + {f_0},{f_y})\quad, $ (2)

where $ {f_x} $ and $ {f_y} $ are spatial frequencies along the x and y axes, respectively. As seen in Eq. (2), the spectra only contain the fundamental frequencies component of object height information.

By filtering the fundamental frequency component of Eq. (2) and performing an inverse Fourier transform, $ h(x,y) $ can be gained, and $ \phi (x,y){\kern 1pt} $ can be recovered. The structural parameters of the measurement system are ${L_0}$ and $d$^[15], when $ {L_0} \gg h(x,y) $ in practical situations, the relationship between $\phi (x,y)$ and $ h(x,y) $ is^[14]

$ \phi (x,y) = - \frac{{2{\text{π}} {f_0}d}}{{{L_0}}}h(x,y)\quad. $ (3)

However, owing to the effects of light illumination, external noise and so on, in real situations, the light intensities of the deformed fringes of the projector systems and that of the CCD input is nonlinear, so the light intensity of the deformed fringe passing through the nonlinear projection system is:

$ g{'}(x,y) = {[g(x,y)]^{\gamma}} = \sum\limits_{k = 0}^\infty {{a_k}\cos \{ k[2{\text{π}} {f_0}x + \phi (x,y)]\} }\quad, $ (4)

where $ {a_k} $ represents the Fourier coefficient, $ k $ is the harmonic component to the order of $ {g{'}}(x,y) $, and $ \gamma $ is the system’s gamma value.

A Fourier transform is executed with Eq. (4). Similarly, after eliminating the zero-order spectrum components in the frequency domain by using the $ {\text{π}} $ phase-shift technology^[14], the frequency domain expression of Eq. (4) becomes:

$ G{'}({f_x},{f_y}) = \sum\limits_{k = 1}^{\infty} {{Q_k}({f_x} - k{f_0},{f_y})} + \sum\limits_{k = 1}^\infty {Q_k^*({f_x} + k{f_0},{f_y})}\quad. $ (5)

It can be seen that when there is a nonlinear relationship in the system, there are higher-order spectra components in the frequency domain of the deformed fringe after the Fourier transform. Mixing the fundamental frequency with higher-order spectra components easily leads to spectra overlapping, which affects the phase measurement accuracy.

The phase information is contained in the fundamental frequency part $ {Q_1}({f_x} - {f_0},{f_y}) $ and $Q_1^* ({f_x} + {f_0},{f_y})$ of the spectra domain. After the low-pass filter is used to filter out the high-order spectra components, almost all of the fundamental frequency components in the frequency domain are obtained, so the nonlinear effect of the system is greatly reduced. The spectra expression gained can be shown as

$ G^\wedge({f_x},{f_y}) = {Q_1}({f_x} - {f_0},{f_y}) + Q_1^*({f_x} + {f_0},{f_y})\quad. $ (6)

2.2 Phase measurement with dual-frequency grating

After the system nonlinear effect is nearly eliminated, the n-th sinusoidal fringe light intensity image outputted by the measurement system is gained through a CCD as follows:

$ \begin{split} g_n^\wedge (x,y) =& {F^{ - 1}}[G^\wedge({f_x},{f_y})] =\\ &\sum\limits_{k = 0}^\infty {A_k^\wedge\cos \{ k[2{\text{π}} {f_0}x + \phi (x,y) + {\delta _n}]\} } \quad, \end{split}$ (7)

where $ {F^{ - 1}}[ \cdot ] $ represents the inverse Fourier transform, $A_k^\wedge$ represents the Fourier coefficient of $g_n^{\wedge}(x,y)$, and $ {\delta _n} $ is the phase-shift amount, $ {\delta _n}{\text{ = }}{{2n{\text{π}} } \mathord{\left/ {\vphantom {{2n{\text{π}} } {{n_1}}}} \right. } {{n_1}}} $, $n = 1,2, \cdots ,{n_1}$.

Using the n-step phase-shift method, the phase gained can be described as

$ {\phi ^ \wedge }(x,y) = {\rm{arc}}{\tan}\left[\frac{{\displaystyle\sum_{n = 1}^N {g^ \wedge _n(x,y)} \sin ({\delta _n})}}{{\displaystyle\sum_{n = 1}^N {g^ \wedge _n(x,y)} \cos ({\delta _n})}}\right] \quad,$ (8)

where $ {\phi ^ \wedge }(x,y) $ represents the wrapped phase.

As $ {\phi ^ \wedge }(x,y) $ is discontinuous, the phase unwrapping must be executed to gain the continuous unwrapped phase $ \phi (x,y) $. Their corresponding relationship is

$ \phi (x,y) = {\phi ^ \wedge }(x,y) + 2k(x,y){\text{π}}\quad, $ (9)

where $ k(x,y) $ is the integer number that represents the fringe orders.

The phase is easy to unwrap by using the low-frequency grating, but this has low phase accuracy. One will gain high phase accuracy by using a high-frequency grating, but the phase is then difficult to unwrap. A dual-frequency grating can make full use of both advantages, so a high-precision recovery phase can be achieved^[16].

When a dual-frequency grating is used to measure phase, Eq. (9) can be further changed into the following expression:

$ \left\{ \begin{gathered} {\phi _{\rm{h}}}(x,y) = {\phi _{\rm{h}}}^ \wedge (x,y) + 2{k_{\rm{h}}}(x,y){\text{π}} \\ {\phi _{\rm{l}}}(x,y) = {\phi _{\rm{l}}}^ \wedge (x,y) + 2{k_{\rm{l}}}(x,y){\text{π}} \\ \end{gathered} \right. \quad,$ (10)

where “ $ {\rm{h}} $” represents high-frequency grating, and “ $ {\rm{l}} $” represents low-frequency grating.

By further changing Eq. (10), it can evolve to Eq. (11) as follows:

$ \begin{split} &{k_{\rm{h}}}(x,y) = \\ &(INT)\left\{ \frac{{{f_{\rm{h}}}}}{{{f_{\rm{l}}}}}{k_{\rm{l}}}(x,y) + \frac{1}{{2{\text{π}} }}\left[\frac{{{f_{\rm{h}}}}}{{{f_l}}}{\phi _{\rm{l}}}^ \wedge (x,y) - {\phi _{\rm{h}}}^ \wedge (x,y)\right]\right\} \quad, \end{split}$ (11)

where $ (INT)\{\cdot\} $ denotes the integer operator, and ${f_{\rm{h}}}$ and ${f_{\rm{l}}}$ represent the fundamental frequency of the high-frequency grating and low-frequency grating, respectively.

So ${k_{\rm{h}}}(x,y){\kern 1pt} {\kern 1pt}$ can be decided by ${k_{\rm{l}}}(x,y){\kern 1pt} {\kern 1pt} $, ${f_{\rm{l}}}$, ${f_{\rm{h}}}$, $ {\phi _{\rm{l}}}^ \wedge (x,y) $ and $ {\phi _{\rm{h}}}^ \wedge (x,y) $. The phase ${\phi _{\rm{h}}}(x,y){\kern 1pt} {\kern 1pt} $ can be gained from Eq. (10), so $ \phi (x,y) $ can be further obtained.

3 Computer simulation and experiment

3.1 Computer simulation

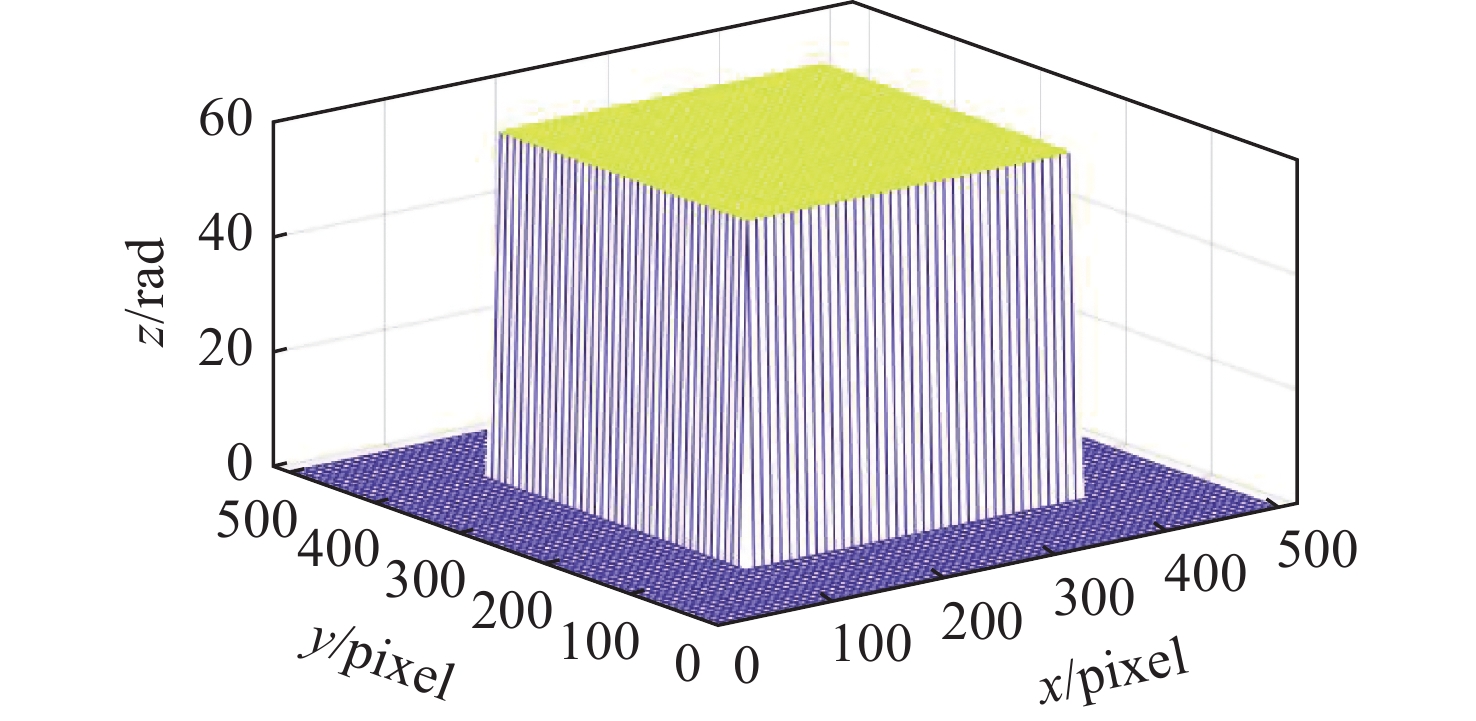
Supposing that the geometric parameters relationship of the phase measurement system is ${{{L_0}} \mathord{\left/ {\vphantom {{{L_0}} d}} \right. } d} = 2.5$, the frequency rate is then ${{{f_{\rm{h}}}} \mathord{\left/ {\vphantom {{{f_h}} {{f_l}}}} \right. } {{f_{\rm{l}}}}} = 4$. The computer-simulated phase is shown in Fig. 1 with a resolution of 512×512 pixels.

There is a nonlinear effect in the system, assuming that $ \gamma {\text{ = }}1.23 $ in Eq. (4). A Fourier transform is executed along the x-axis aimed to the light intensity of the deformed fringe. The $ {\text{π}} $ phase-shift technique^[14] is applied to eliminate the zero-order spectrum component of the frequency domain. The spectra distributions gained are shown in Fig. 2 (a) It can be seen that the spectra distributions contain high-order spectra components. After the nonlinear influence of the system is nearly eliminated, the results have nearly no high-order spectra components as shown in Fig. 2 (b). On the basis of there being nearly no nonlinear effect, the spectra distribution obtained by the dual-frequency grating method contains only the fundamental frequency components of the two gratings, as shown in Fig. 2 (c).

图 2.

Fig. 2. Spectra distributions along x axis

下载图片 查看所有图片

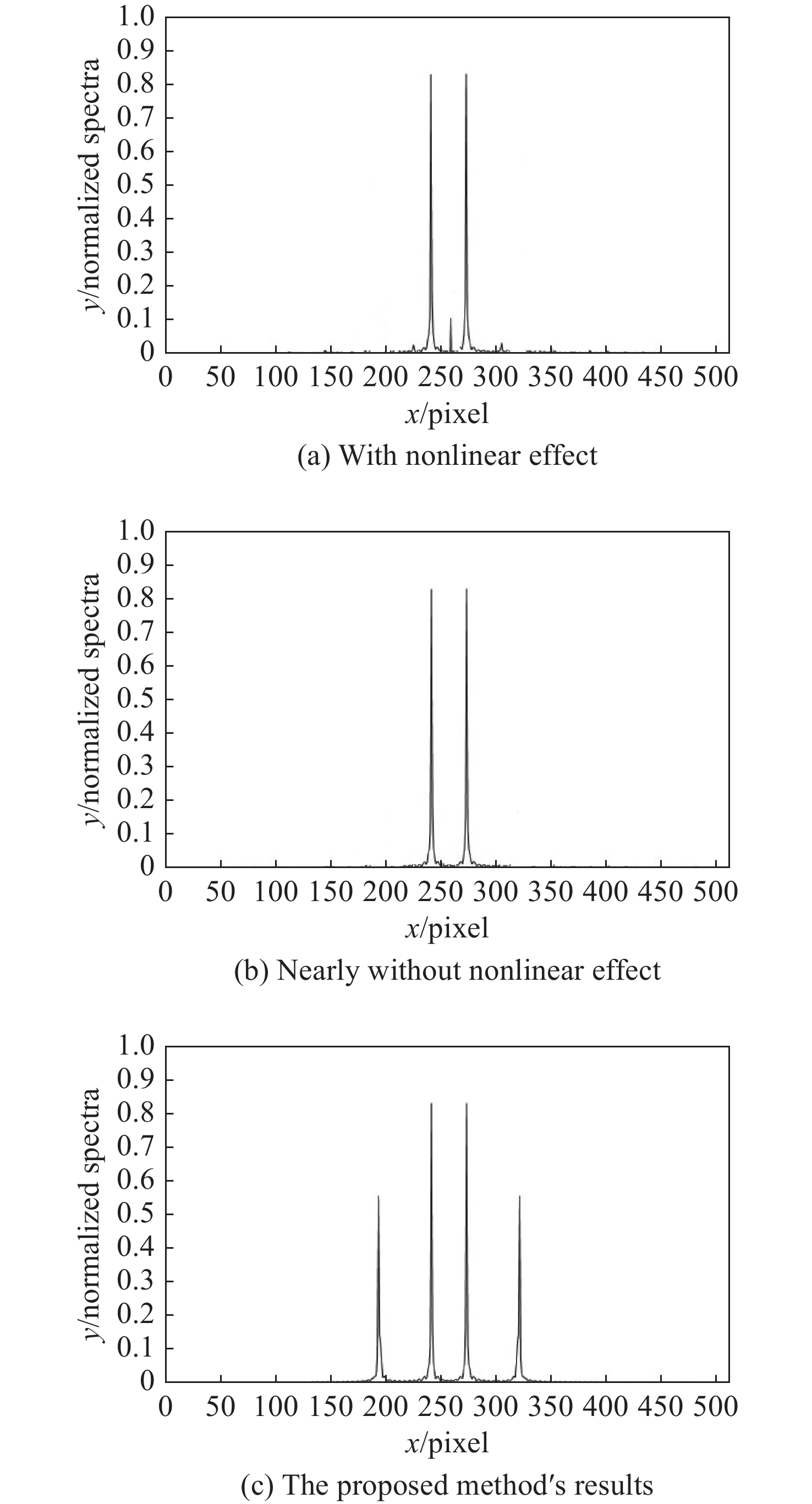
After applying the inverse Fourier transform to these spectra distributions as shown in Fig.2, the gained measurement errors between the recovery phase and the original phase by using the three methods as shown in Figs. 3 (a)−(c) (color online), respectively.

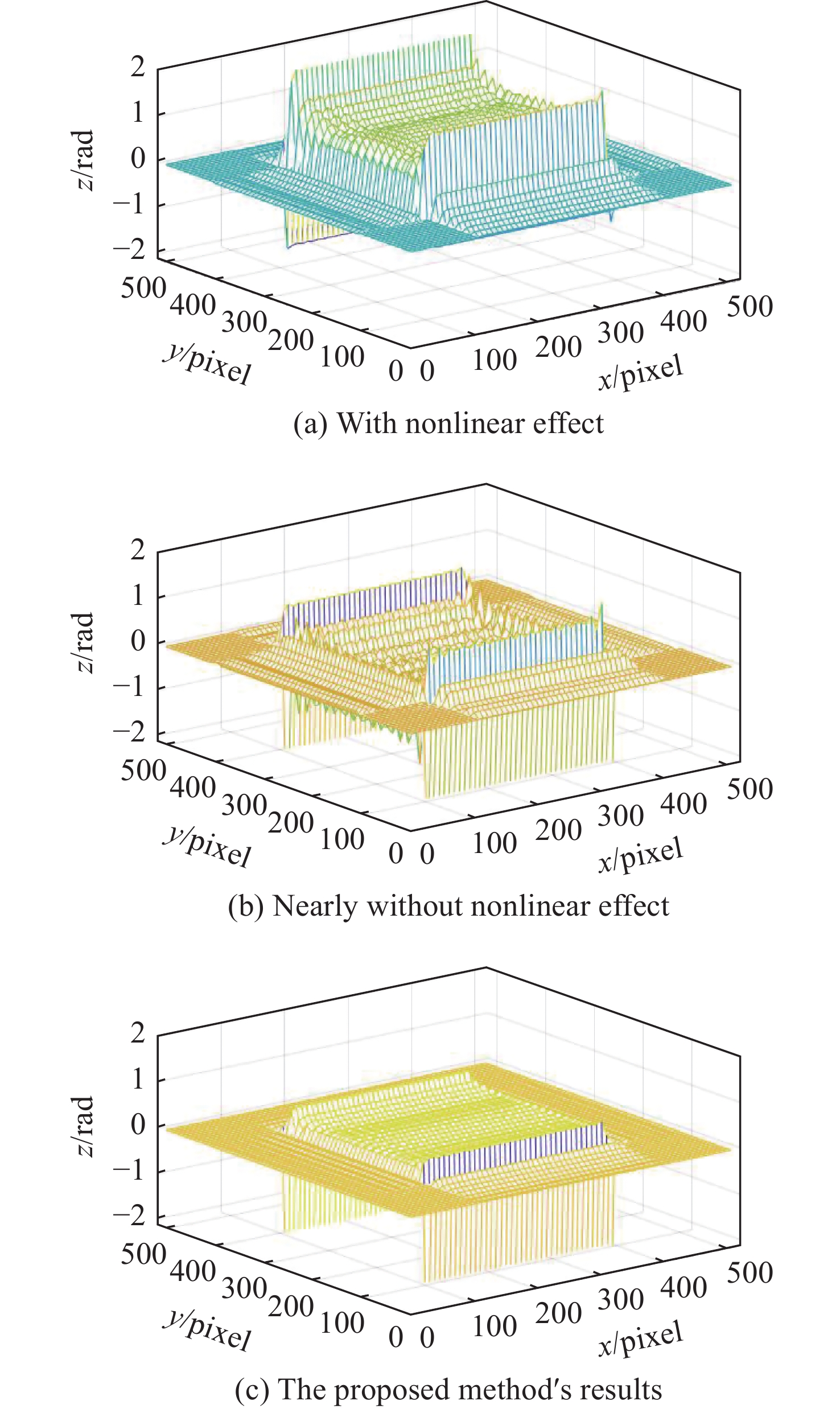
图 3.

Fig. 3. Phase measurement error diagrams gained by three different simulation methods

下载图片 查看所有图片

The average phase error value of Fig. 3 (a), 3(b) and 3(c) is 0.8452 rad, 0.4438 rad and 0.2364 rad, respectively. The error value of this method is 27.97% and 52.51% that of the method with a nonlinear effect and nearly completely without nonlinear influence, respectively.

The phase in the nonlinear measurement system can be recovered effectively by using the proposed method and its phase recovery error is the smallest among the three methods.

3.2 Actual experiment

To further prove the correctness and feasibility of the principle analysis, the actual experiment was executed by using the experimental system shown in Fig. 4. Through a program generated by MATLAB software, ${{{f_{\rm{h}}}} \mathord{\left/ {\vphantom {{{f_h}} {{f_l}}}} \right. } {{f_{\rm{l}}}}} = 4$ can be gained.

图 4.

Fig. 4. The structural frame for the experimental device

下载图片 查看所有图片

The experimental model used is a steep stepped object, as shown in Fig. 5 (color online).

图 5.

Fig. 5. The experiment model used in the experiment

下载图片 查看所有图片

In the experiment, the same three methods of computer simulation are used to measure the phase. The recovery results gained can be shown in Fig. 6 (a), 6(b) and 6(c) (color online), respectively. Fig. 6 (d) is the cross-section comparison results of 100th column of Fig. 6 (a) ~ (c), respectively.

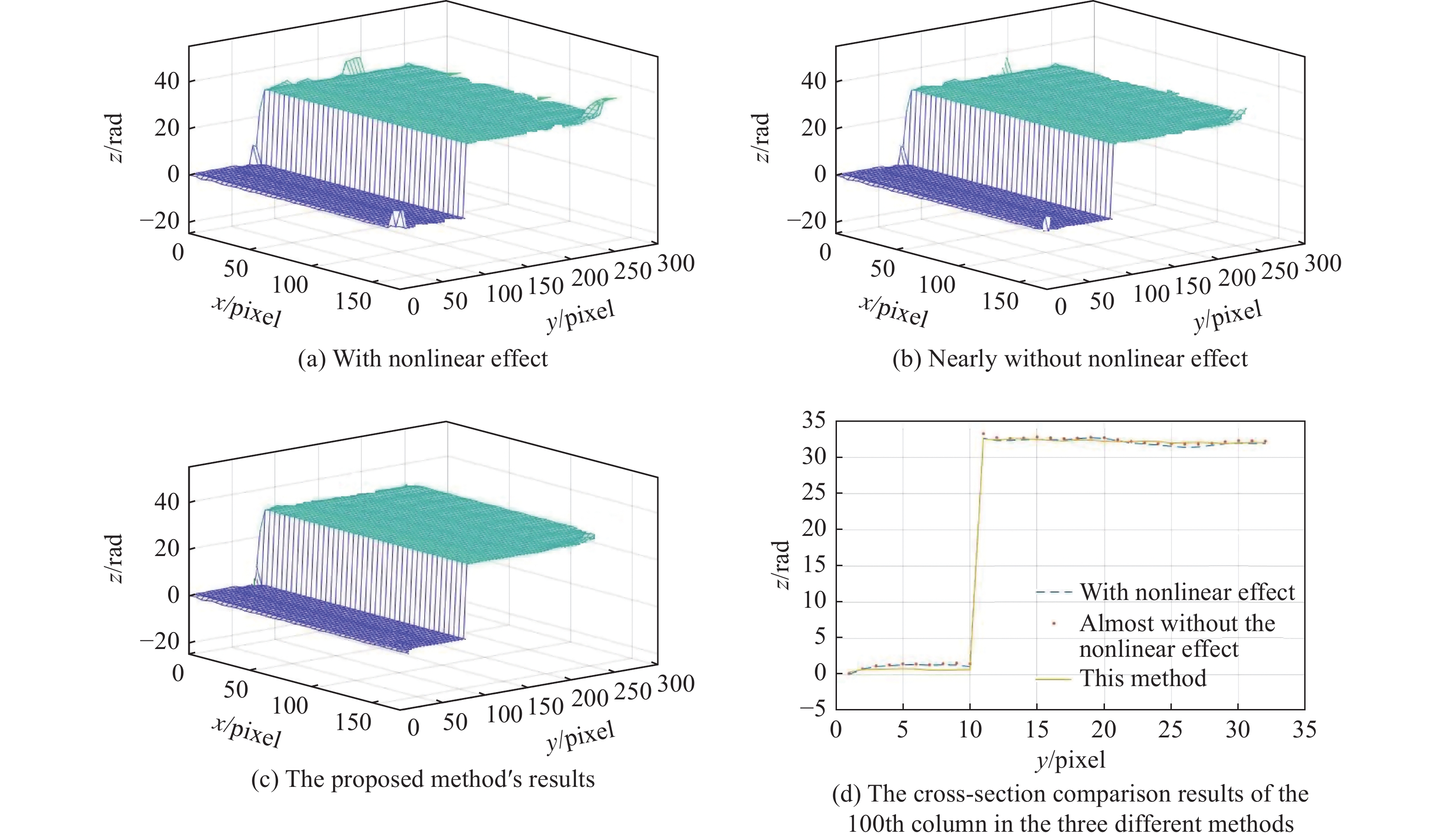
图 6.

Fig. 6. Phase measurement results gained by using the three different experiment methods

下载图片 查看所有图片

It indicate that the phase measurement result using the method proposed in this paper is best, and the gained phase surface contour is complete and smooth.

4 Conclusion

Due to the influence of the nonlinear effect on phase measurement, the influence of the nonlinear effect on fringe intensity in the measurement system is analyzed. The dual-frequency grating method is used to improve the phase measurement accuracy, and the principle is analyzed.

To verify the effectiveness of the proposed principle analysis, the computer simulation and practical experiment are implemented, and the results gained are consistent with the principle analysis. The error values of this method by simulation are 27.97% and 52.51% of the methods with and nearly without nonlinear influence, respectively, showing that the effect of phase recovery is the best among those in the experiment. This demonstrates that the phase measurement method proposed in this paper is effective and feasible.